Regression IIII

Lecture: …

Dr. Elijah Meyer

NC State University
ST 511 - Fall 2024

2024-11-13

Checklist

– Keep up with Slack

– HW 4 (late window tonight 11:59)

– HW 5 has been released (due Sunday at 11:59)

– Quiz 10 (released Wednesday; due Sunday Nov 10)

– Download today’s AE

HW-3

– Grades are posted (check after class)

– Key coming out tonight

– Regrade window will be announced on Slack

Homework schedule

Overlapping homework are not idea, but it gives you flexibility on when to complete assignments

– Homework 5 due Tuesday (Nov 19th at 11:59)

– Homework 6 assigned Monday (Nov 18; due Tuesday Nov 26)

This gives more time + an additional office hour for Homework 5 (if needed)

Class check-in

Reported difficult concepts

– ANOVA, Chi-square, Tukey HSD, the distributions, as well as the degree-of-freedom for the distributions

– which method should be used for different scenarios/data sets

– difference between p-values and confidence intervals

– difference between theory and simulation scenarios

Last Time

\(\widehat{\text{flipper length}} = 147.563 + 1.10*\text{bill length}\) \(- 5.25*\text{Chinstrap} + 17.55*\text{Gentoo}\)

\[\begin{cases} 1 & \text{if Chinstrap level}\\ 0 & \text{else} \end{cases}\] \[\begin{cases} 1 & \text{if Gentoo level}\\ 0 & \text{else} \end{cases}\]

Quantitative explanatory variables

flipper length ~ body mass + bill length

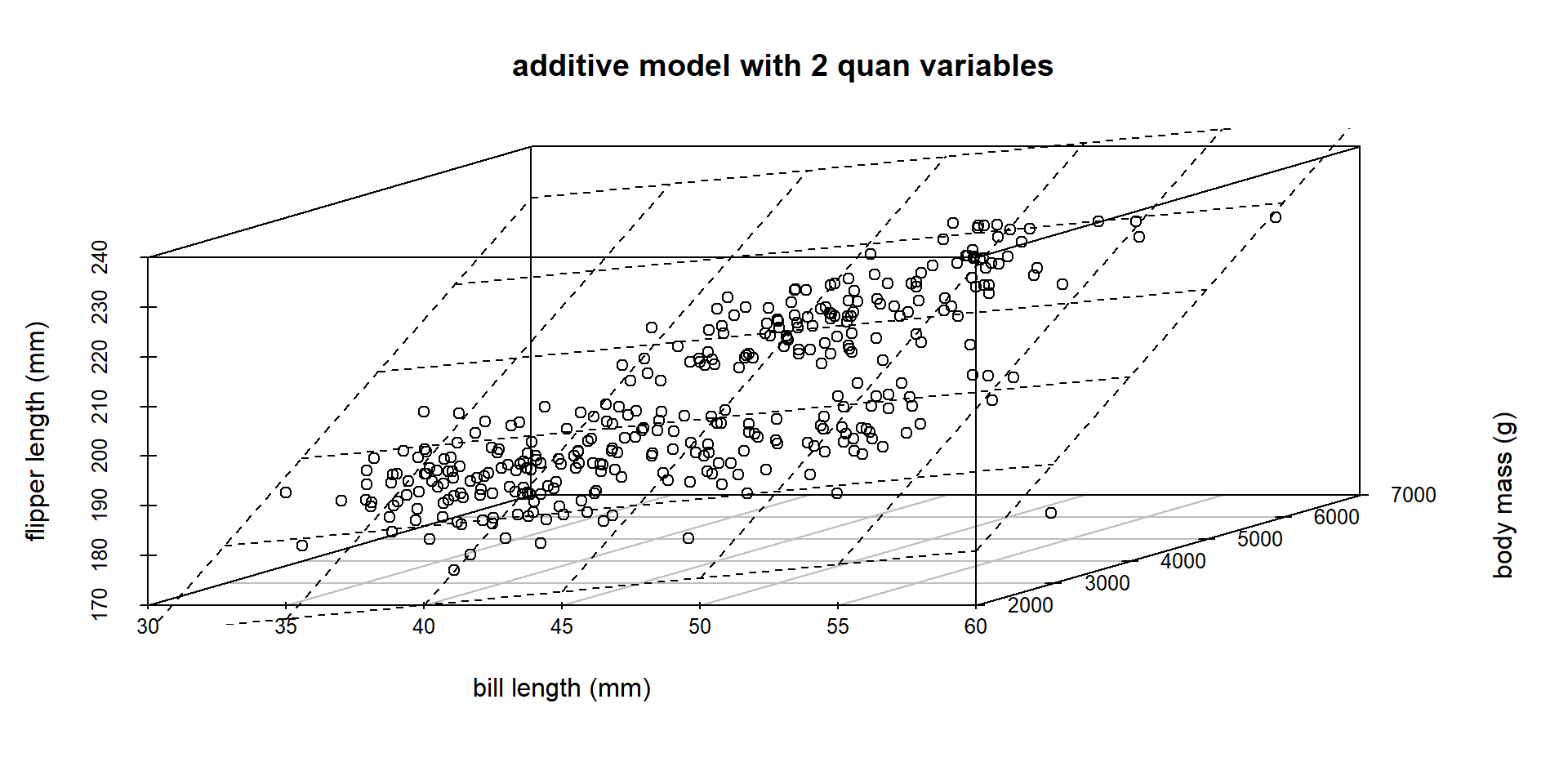
Model Output

How do we interpret body mass in the context of the problem?

round(summary(model2)$coefficients,3)
               Estimate Std. Error t value Pr(>|t|)
(Intercept)     121.956      2.855  42.715        0
bill_length_mm    0.549      0.080   6.859        0
body_mass_g       0.013      0.001  23.939        0

AE Interaction Model

Which model should we fit?

Model Selection

We can start with visual evidence (what does it look like we should fit?)

Follow up with more statistical evidence:

  > AIC (8.4)

  > BIC (8.4)

  > Adjusted R-squared (8.3) <- what we will cover

Visual evidence

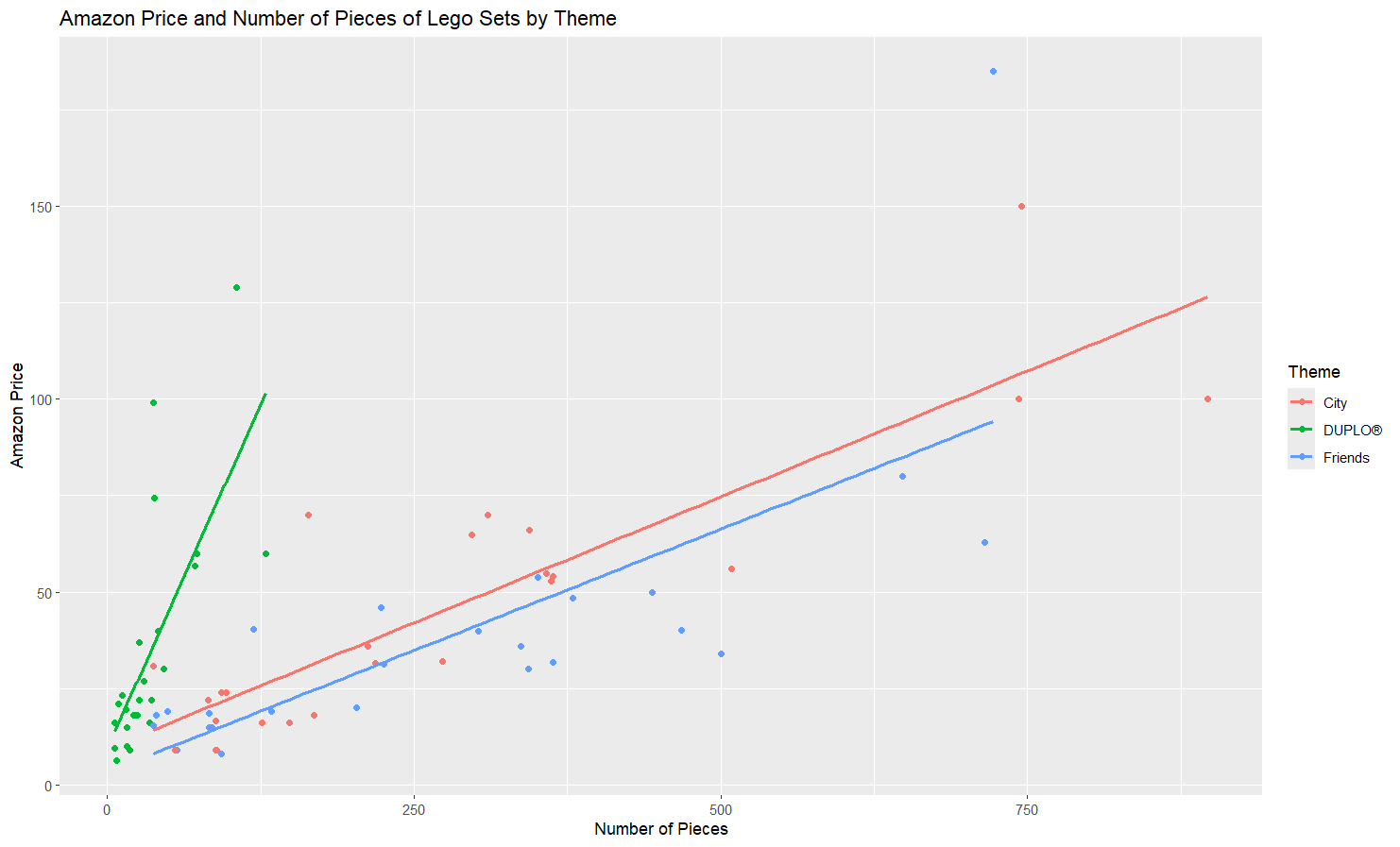
Visual evidence

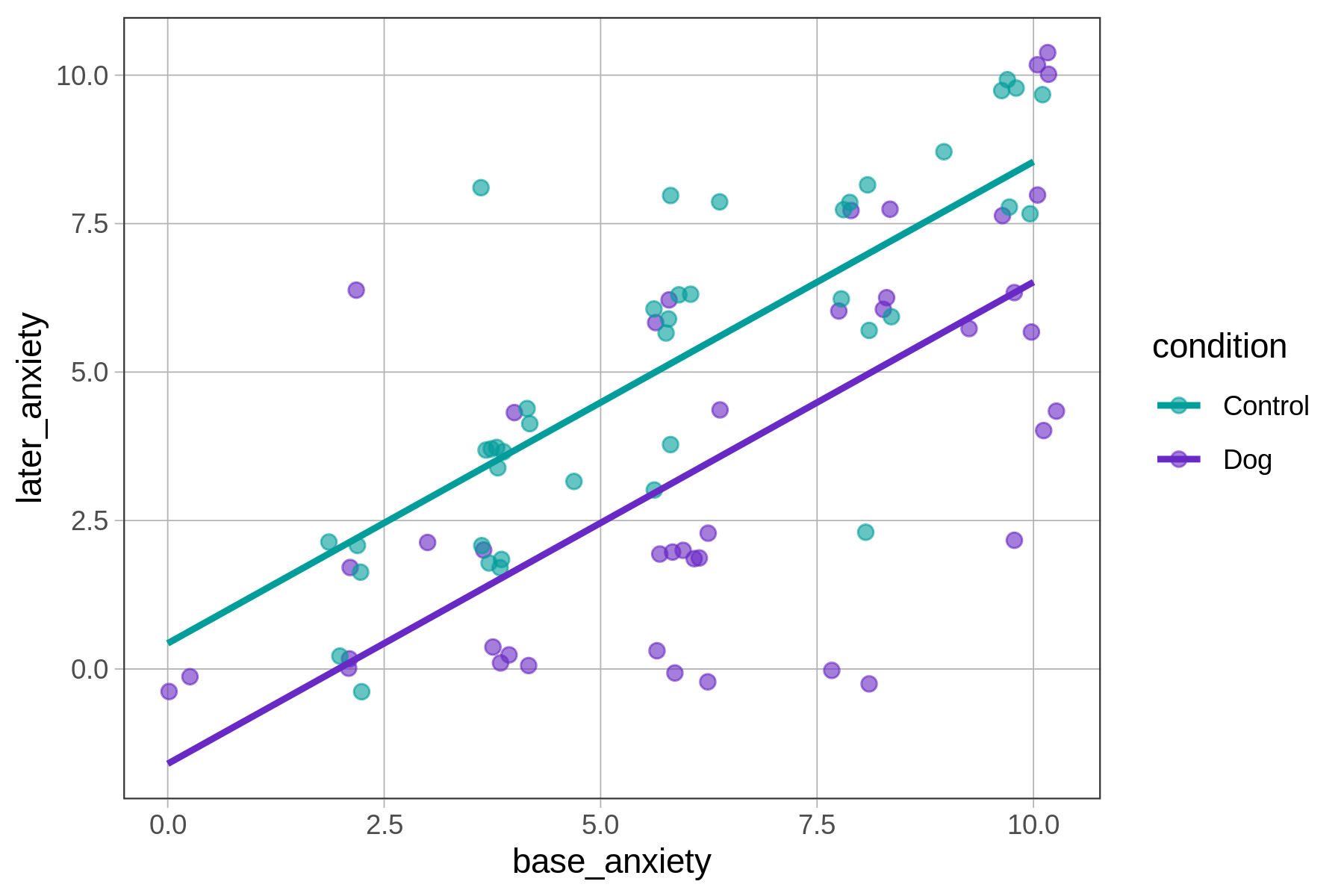
Model Selection

Adjusted R-squared

Adjusted R-squared is a modified version of R-squared that has been adjusted for the number of predictors in the model.

Formula

The \(\frac{n-1}{n-k-1}\) acts as a “penalty” based on the number of predictors (k)

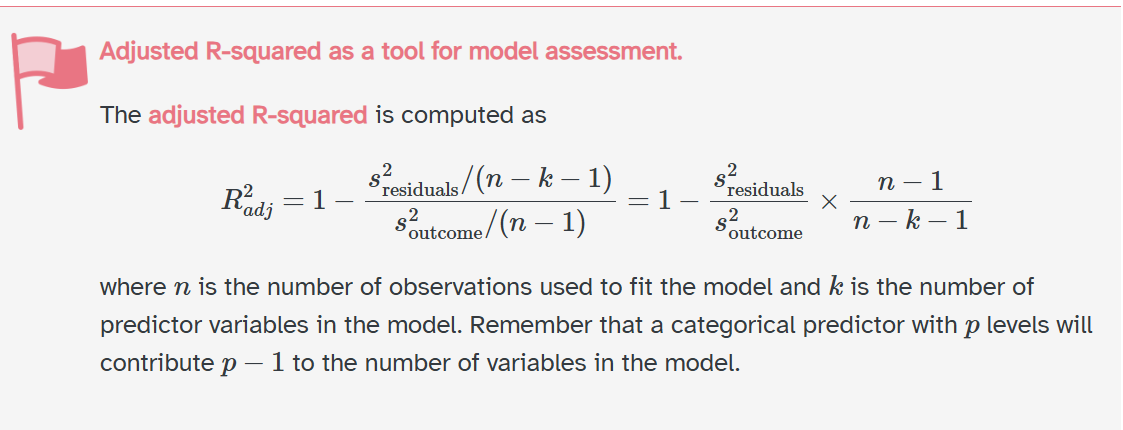
Takeaway: If you add a useful predictor, adjusted \(R^2\) will still increase because the \(s^2 \text{residuals}\) will go down larger than the penalty.

If you add a predictor that is not useful, adjusted \(R^2\) will decrease because of the \(n-k-1\).

🔗 class website